How Do Map Projections Influence the Portrayal of Spatial Data?

EARTH IS NOT FLAT, so a flat map cannot portray all locations accurately. An ideal map would preserve directions, distances, shapes, and areas, but it is not possible to preserve all four of these accurately. Instead, either the shape of features on a map, such as country outlines, is preserved or the area of features is preserved, but never both at the same time. Many map projections depict both shape and area somewhat inaccurately, as a trade-off, so that neither will be shown more inaccurately. Cartographers (map makers) have developed different ways of projecting our three-dimensional world onto a flat map, and each approach is called a map projection. The particular type of projection is chosen based on the intended use of the map.

What Is the Rationale Behind Map Projections?

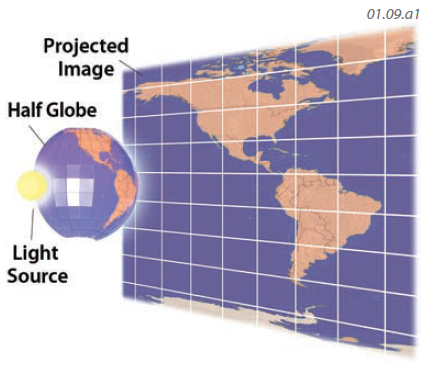
A map projection is a mathematical algorithm used to represent places on a three-dimensional spherical Earth onto a flat map. Imagine shining a light through a partially transparent globe and observing the image projected on the back wall. This is what a map projection does, but in a quantitative, numeric way. While many projections exist, the best projection for a given map will introduce the least distortion for the key areas being shown. Whenever a map is made, some distortion is introduced by the projection. It is impossible to avoid distorting either shapes or areas, or doing some distortions of each.

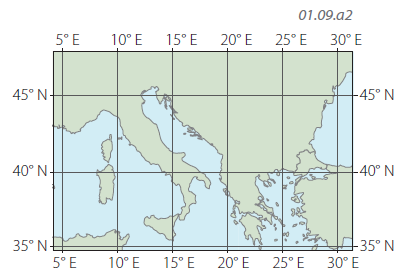
Some map projections attempt to preserve shapes, and are called conformal. If shapes are preserved, directions may be preserved but areas are distorted and scale will vary across the map. These imperfections get worse for maps that show larger areas.

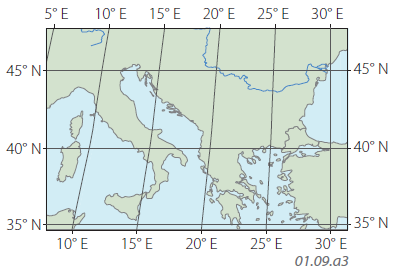
In equal-area projections, areas are preserved but shape is distorted. Compass directions cannot be shown correctly, so such a map should not be used for navigation. If the proper projection is selected for a given application, distortions are minimized for the aspect (e.g., shape) that is most important and for the region of most interest.

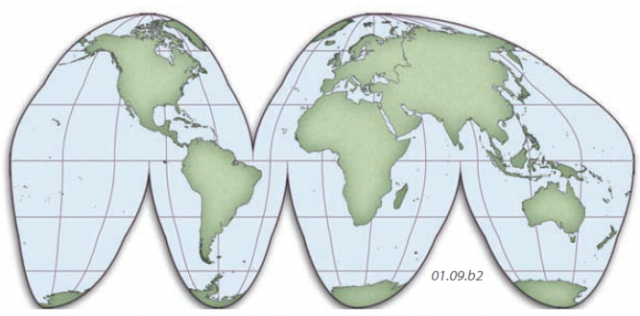
What Are the Major Types of Projections and What Advantages Does Each Offer?

Sinusoidal

Perhaps the easiest projection to visualize conceptually is to imagine peeling an orange and slicing it in a few strategic places to allow it to be flattened without buckling. Sinusoidal projections work on this same premise. If the map can be interrupted so that areas of lesser significance for a given application are not shown, then less distortion exists in the areas that are shown. Straight, parallel lines remain so, and have their correct length. Meridians become progressively longer toward the edges of each lobe of the map. While areas are preserved, shape distortion increases near the edges of each lobe.

The shape distortion problem in such projections can be mitigated by increasing the number of central meridians around which accuracy is preserved. However, this comes at the expense of having more areas of interrupted coverage. Notice how the central meridians are straightest and appear at right angles to the parallels at the equator. These are the areas that are depicted most accurately for this type of projection. The most common type of map using this projection strategy is called a Goode projection.

Cylindrical

In cylindrical projections, the globe is transformed to a flat page by projecting a globe outward onto a cylinder. The projection starts at a line, called the standard line, where the globe touches the cylinder, usually at the equator. These types of map projections have no distortion at the standard line (equator), but distortion becomes worse with increasing distance from the standard line. The resulting maps portray parallels of latitude as straight lines with the same length as the equator (that is, distorted in length) and depicts meridians also as straight lines intersecting the parallels at right angles.

Cylindrical projections depict compass directions as straight lines, so they are excellent for navigation. However, because the meridians are depicted (falsely) as being parallel to each other, east-west exaggeration of distances is severe, particularly in high latitudes. To allow these maps to be conformal (preserve shapes), north-south distances are stretched to match the east-west exaggeration. This makes high-latitude areas greatly exaggerated in size, but they retain shapes.

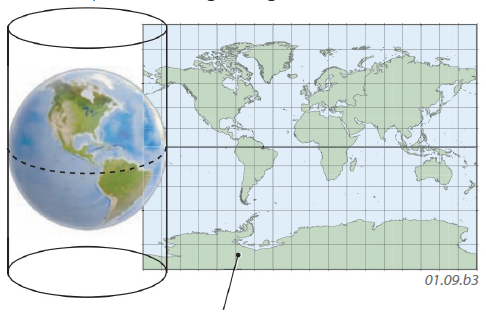
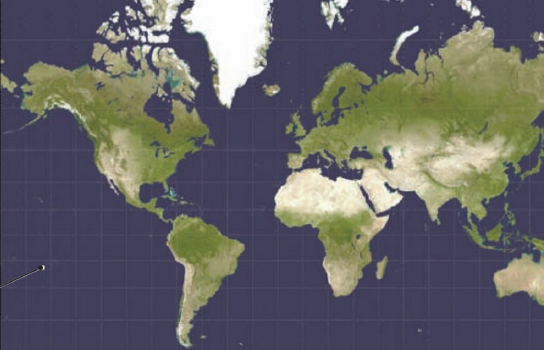
The most familiar type of cylindrical projection is the Mercator map, which became an important tool in the Age of Exploration. The part of the map at the right, which is a Mercator projection, portrays Greenland as being larger than the conterminous U.S. Is this true?

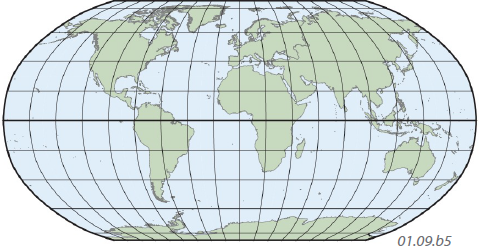
Some maps blend aspects of a cylindrical map with other types of projections. The Robinson projection is a commonly used projection of a world map, especially in textbooks. It does not fully preserve areas or shapes, instead representing a compromise between equal-area and conformal projections. The meridians curve gently, and the parallels are straight lines horizontally across the map. A feature it shares with cylindrical maps is severe distortion near the poles.

Conical

Conical projections involve conceptualizing a cone over the globe, usually with the apex of the cone vertically above the pole. No distortion occurs along the arc where the globe touches the cone— the standard line, usually a parallel of latitude. If the cone slices through the globe and intersects the surface along two arcs (usually parallels of latitude), the projection is called polyconical. In either case, distortion increases with distance away from these arcs.

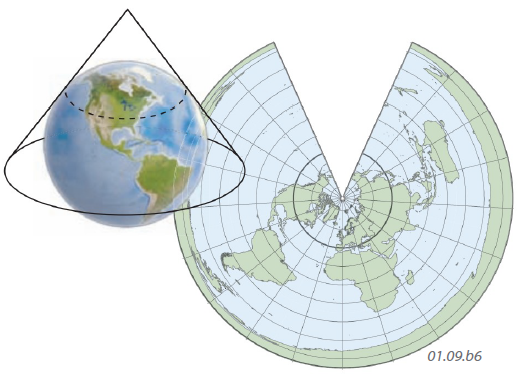
In conical projections, parallels are concentric circles and meridians are lines radiating from the center of curvature of the parallels. This family of projections is neither equal area nor conformal.

Conical projections can only show areas within a single, complete hemisphere (since regions that curve underneath the globe cannot be projected). This type of map works best when the area mapped is small in latitudinal extent. Notice how poorly this projection performs for showing a large area.

Planar

In planar projections, the plane onto which the map is projected touches the globe in a single point, which becomes the center of the map. Distortion increases away from this point, and any straight line from this point is a line of true direction. Again, only one full hemisphere can be shown on such a map.

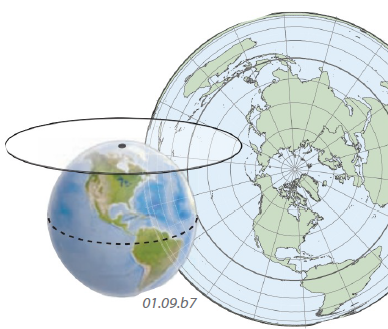
The pole is the focus in the polar stereographic projection. Scale becomes exaggerated toward the equator, but all lines connecting the shortest distance between two points on the sphere (great circles) are shown as straight lines. Planar projections are useful, therefore, for air navigation.